Since the discovery in 1772 of the stable Lagrange points L4 and L5 (Figure 1) in the gravitational field of two massive bodies moving under the sole influence of their mutual gravitational forces it is known that in these points a small object affected only by the gravitational forces of the two large masses will maintain its position relative to them. Astronomers found a large number of minor celestial bodies around the L4 and L5 points of the Sun-Jupiter, Sun-Earth, Sun-Mars and Sun-Neptune systems. In 1961, the Polish astronomer, Kazimierz Kordylewski had observed two bright patches near the L5 point of the Earth-Moon system with photography, and since that time this formation is called the Kordylewski dust cloud (KDC). However many astronomers assume that these dust clouds do not exist, because the gravitational perturbation of the Sun, solar wind and other planets may disrupt the stabilizing effect of the L4 and L5 points of the Earth and Moon. Using ground-born imaging polarimetry, Hungarian researchers found new observational evidence for the existence of the KDC around the L5 point of the Earth-Moon system. Excluding all possible artefacts induced by the telescope or the Earth’s atmosphere, the only explanation remains the polarized scattering of sunlight on the particles collected around the L5 point. By their polarimetric detection of the KDC the researchers think it is appropriate to reconsider the pioneering photometric observation of Kordylewski. The new polarimetric evidence is also supported by the results of computer simulations of dust cloud formation in the L5 point of the Earth-Moon system.
Many examples demonstrate well that polarimetry is a useful technique to gather astronomical information from spatially extended phenomena. Telescopes mounted with polarizers can study the neutral points of the Earth’s atmosphere, the solar corona, the surface of planets and moons of the Solar System, distant stars, galaxies and nebulae. In astronomy, the majority of knowledge originates from information obtained via light. The light that we receive directly from the Sun is unpolarized, but when it is scattered by dust or atmosphere, it will be linearly polarized. Furthermore, since longer wavelengths are scattered less than shorter ones and the Kordylewski dust cloud (KDC) can be detected by dust-scattered light (Figure 2A,B), the photometric detection of the KDC is even harder in the infrared than in the visible spectral range. But in both ranges there is a very faint light and the KDC can be visually detected only from small phase angles (determined by the observer, the Sun and the L4/L5 point), i.e. at or near ’full dust moon’. However, if linearly polarized light is detected, then the degree of polarization p of dust-scattered sunlight is maximal at phase angles near to 90o.
“Indeed, at phase angles approximating 90o, we successfully detected the polarization signature of the KDC in the L5 point of the Earth and Moon with imaging polarimetry. Theoretically, dust-scattered sunlight becomes partially linearly polarized with the direction of polarization perpendicular to the scattering plane determined by the Sun, the ground-born observer and the dust region observed. We have indeed found this forecasted polarization characteristics in the patterns of the angle of polarization α (Figure 2B). This is one of the strongest proof that we observed a sunlit light-scattering object outside the Earth’s atmosphere, rather than a terrestrial phenomenon. A further fact supporting our observation of the KDC is that in the measured α-patterns several clusters occur (Figure 2B), as our computer simulations also suggest (Figure 2C).” – explained Professor Gábor Horváth, head of the Environmental Optics Laboratory of Eötvös University in Budapest, who led the study, the results of which have been recently published by the Monthly Notices of the Royal Astronomical Society in a two-part paper [1, 2]:
[1] Judit Slíz-Balogh, András Barta, Gábor Horváth (2018) Celestial mechanics and polarization optics of the Kordylewski dust cloud in the Earth-Moon Lagrange point L5 - Part I. Three-dimensional celestial mechanical modelling of dust cloud formation. Monthly Notices of the Royal Astronomical Society 480 (4): 5550-5559 (doi: 10.1093/mnras/sty2049)
[2] Judit Slíz-Balogh, András Barta, Gábor Horváth (2018) Celestial mechanics and polarization optics of the Kordylewski dust cloud in the Earth-Moon Lagrange point L5 - Part II. Imaging polarimetric observation: new evidence for the existence of Kordylewski dust cloud. Monthly Notices of the Royal Astronomical Society 482 (1): 762-770 (doi: 10.1093/mnras/sty2630)
”Our imaging polarimetric measurements have been performed by a cooled CCD camera mounted with three linearly polarizing filters, the transmission axis of which was rotated by 120o relative to each other. At the chosen celestial region, we took one photograph through each polarizing filter with an exposure of 180 seconds.” – said Judit Slíz, an astronomer of the Eötvös University, who performed the measurements and observations.
”To detect the KDC, we used sequential imaging polarimetry: After taking three photographs of the selected sky area at night through a linearly polarizing filter with three different directions of polarization, we evaluated these polarization pictures with our self-developed image-processing software called Algonet®. Finally, we obtained the patterns of the radiance, degree and angle of polarization of the observed celestial region in the red, green and blue spectral ranges.” – said physicist dr. András Barta, head of the Estrato Research and Development Ldt. in Budapest.
Figures 3 and 4 illustrate the starry night sky with the Earth, Moon, Sun and the Kordylewski dust cloud at the L5 Lagrange point of the Earth-Moon system, if the dust cloud were visible with the naked eye.
The Hungarian scientists have also investigated a three-dimensional barycentric four-body computer model consisting of the Sun, Earth, Moon and one test particle, 1860000 times separately. They mapped the size and shape of the cluster of particles not escaped from the system sooner than an integration time of 3650 days around the Lagrange point L5 of the Earth and Moon (Figure 2C). Apart from the gravitational force, the forces from the radial solar radiation pressure and the Poynting-Robertson drag was also investigated, but they were negliglibe. The computer simulation showed that objects ranging from micron to rock size can circulate along with the Lagrange point L5 for a long time.
”In special cases, there are exact well-known classic analytical solutions of the gravitational three-body problem. However, the three-dimensional four-body (Sun, Earth, Moon, test particle) problem can be solved only numerically. Our computational method was an adaptive step size Runge-Kutta-Fehlberg integrator. We were curious whether there is any chance of finding material at the Lagrange points L4 and L5. The results of our simulation showed that these Lagrange points can trap many particles for several years, but this particle cloud has a continuously changing, pulsing and whirling shape (Figure 2C).” – said Judit Slíz, who also performed the computer simulations.
The stability of the L4 and L5 Lagrange points of the Earth and Moon has some well-exploitable advantages: They are suitable for spacecraft, satellite or space telescope parking with minimal fuel consumption (nonetheless at the moment there are no spacecraft orbiting neither at L4 nor at L5 in the Solar System), or they can be used as transfer stations for the mission to Mars or other planets, and/or to the interplanetary superhighway. It has also been suggested that the unwanted carbon-dioxide amounts extracted from the Earth’s atmosphere could be lauched to the L4 and L5 points of the Earth-Moon system in order to dispose this dangerous gas with strong greenhouse effect. The investigation of the dynamics of the KDC is also important from the point of view of space navigation safety.
More: press release by the Royal Astronomical Society
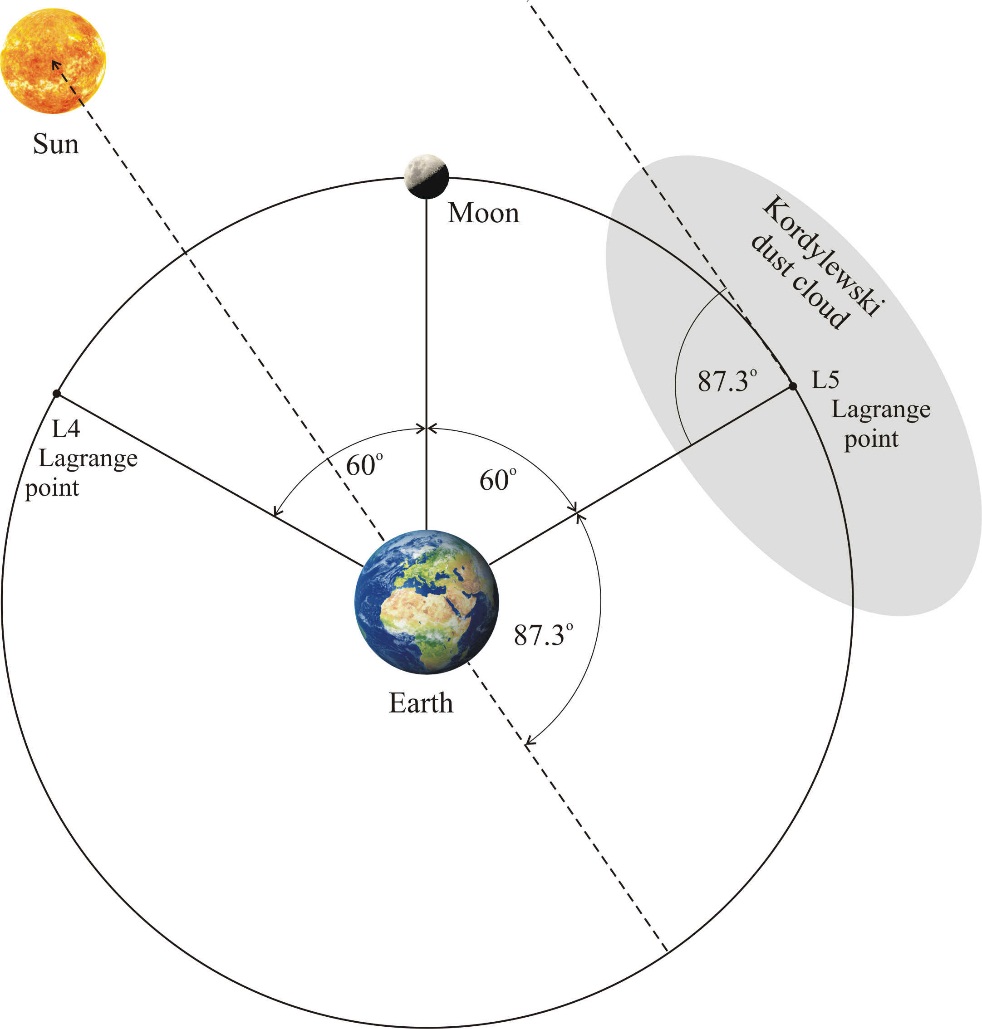
Figure 1: Positions of the Moon and the L5 Lagrange point of the Earth-Moon system in the plane of the Moon’s orbit on 19 August 2017 at 01:14:15 UT with 87.3o phase angle. Apart from the Earth and Moon, the relative dimensions are not to scale. The Sun's direction is indicated by an arrow.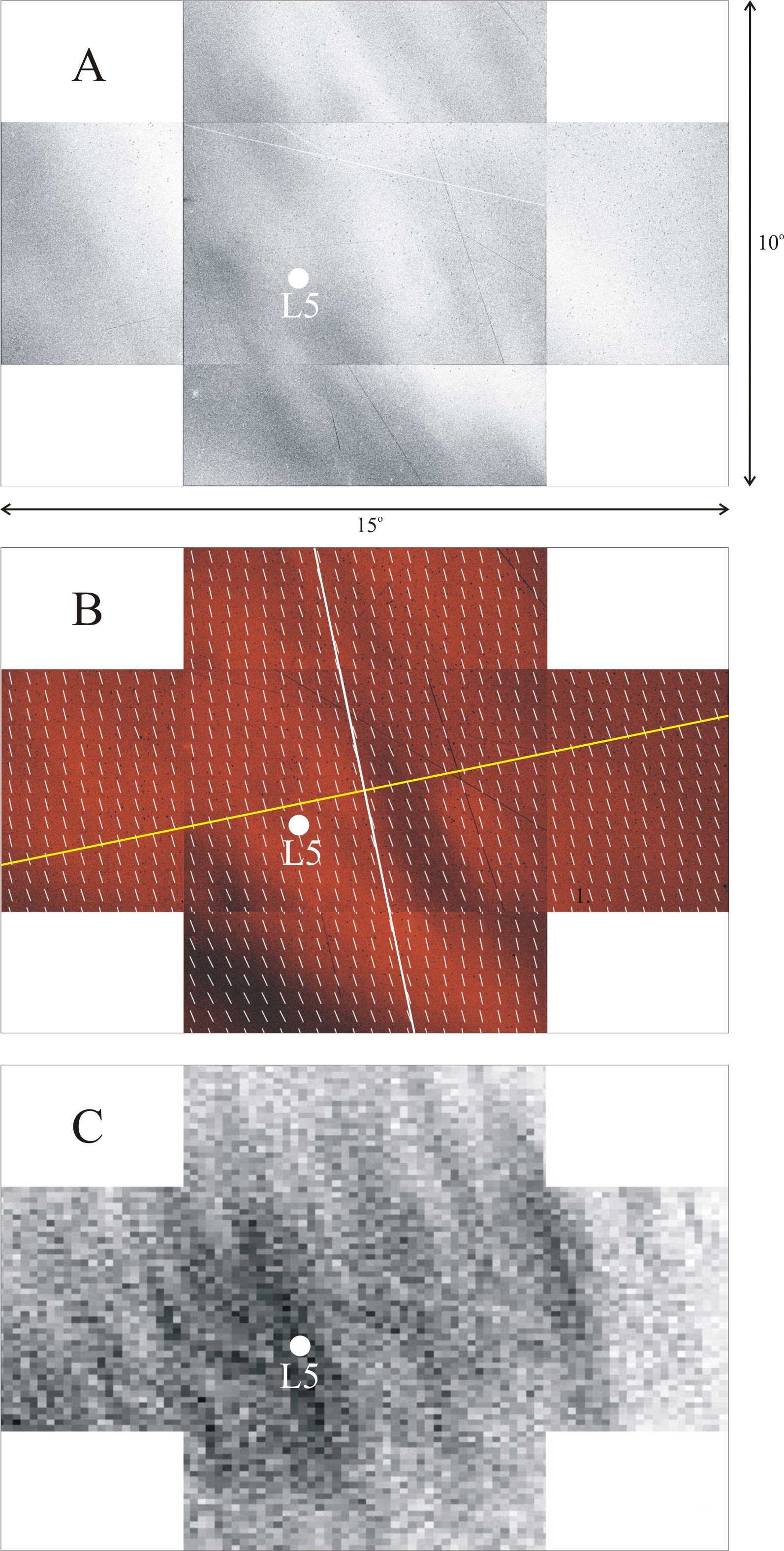
Figure 2: (A, B) Mosaic pattern of the measured degree of linear polarization p (A) and angle of polarization α (clockwise from the scattering plane) of the Kordylewski dust cloud around the L5 point (dot) of the Earth-Moon system measured on 19 August 2017 in the green (550 nm) spectral range with imaging polarimetry. The short white bars represent the local directions of polarization. The long yellow and white straight lines show the scattering plane and the perpendicular plane passing through the center of the picture, respectively. (C) Computer-simulated volume density distribution of the particles of the Kordylewski dust cloud around the L5 point (white dot) of the Earth-Moon system.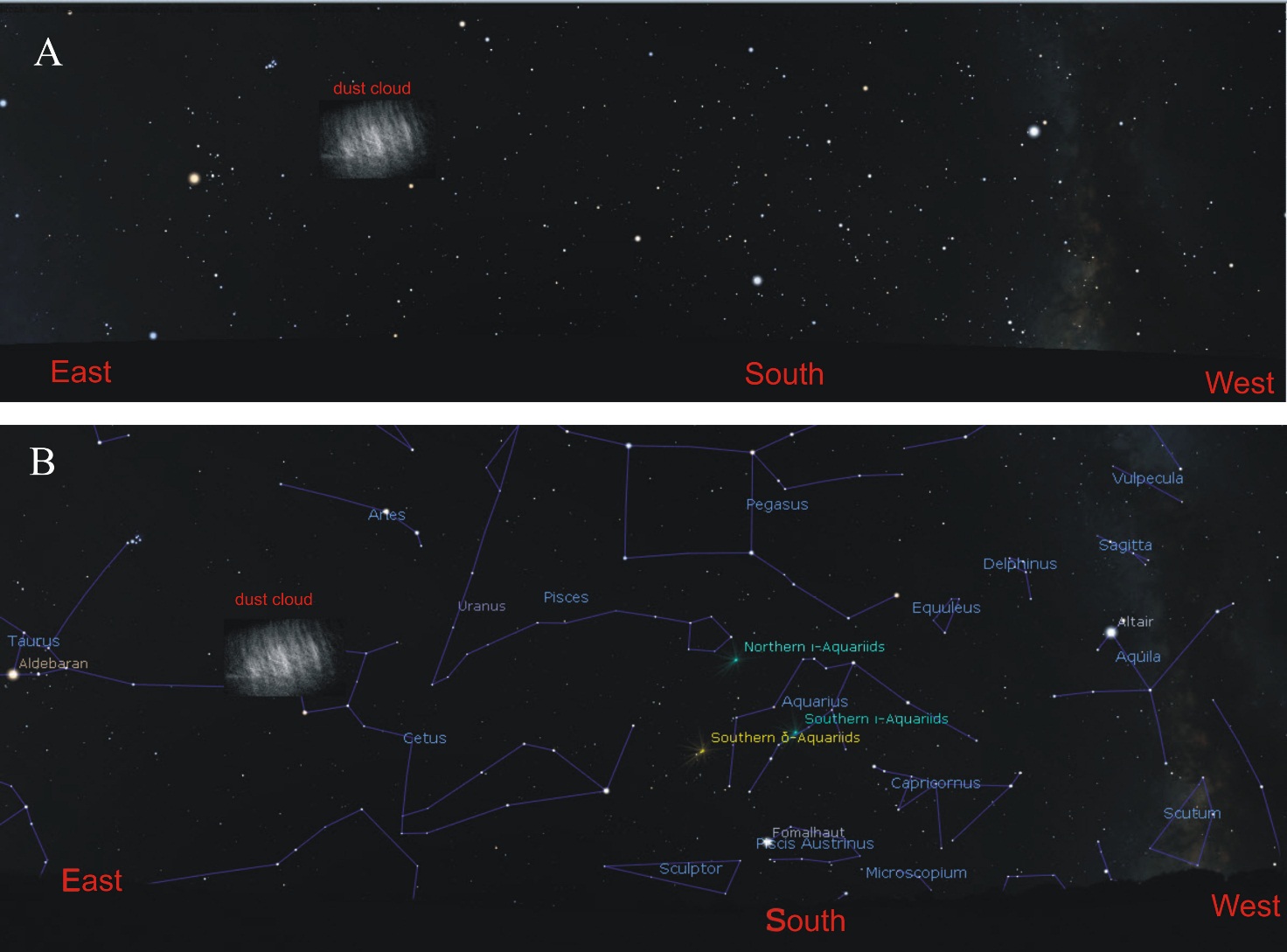
Figure 3: (A) So would look the Kordylewski dust cloud around the L5 Lagrange point of the Earth-Moon system on the night sky at 01:14 UT on 19 August 2017 at the time of its imaging polarimetric measurement and computer modelling, if we could see it with the naked eye. (B) As Fig. A with indication of the most famous star constellations and their names.
Figure 4: Illustration (not to scale) of the starry night sky with the Earth, Moon, Sun and the Kordylewski dust cloud at the L5 Lagrange point of the Earth-Moon system, if the dust cloud were visible with the naked eye.






